Mesh Boolean¶
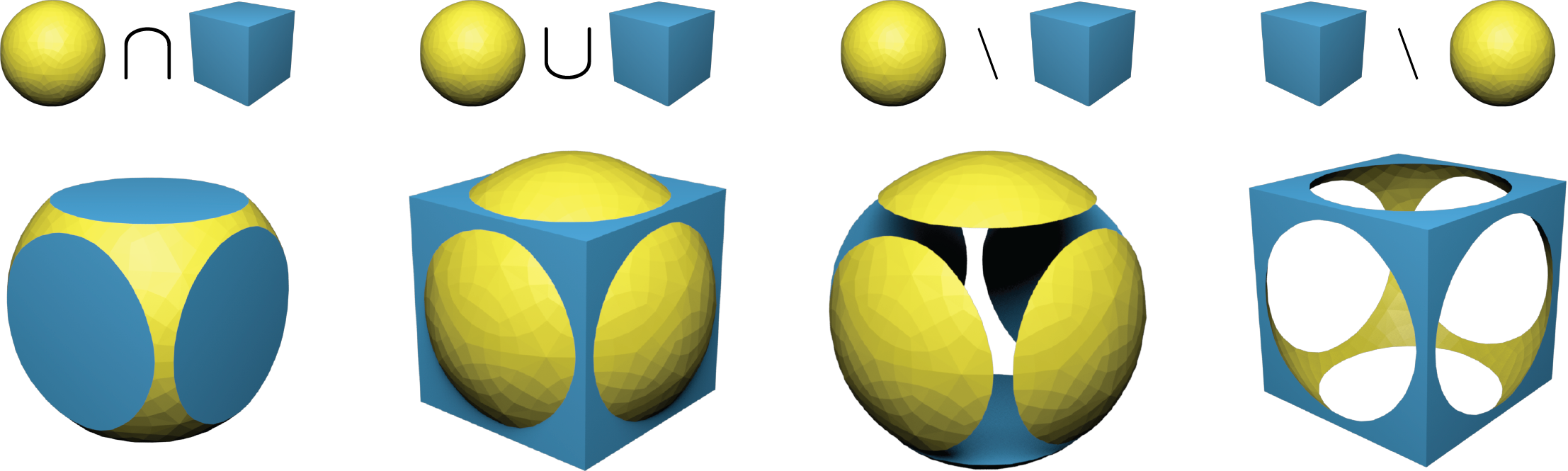
Boolean operation is one of the fundamental operations for 3D modeling. It combines two or more solid shapes (say \(A\) and \(B\)) by checking if a point \(x\) lies inside of each solid. Four commonly used binary boolean operations are:
- Union: \(A \cup B := \{ x \in \mathbb{R}^3 \mid x \in A \textrm{ and } x \in B \}\)
- Intersection: \(A \cap B := \{ x \in \mathbb{R}^3 \mid x \in A \textrm{ or } x \in B \}\)
- Difference: \(A \setminus B := \{ x \in \mathbb{R}^3 \mid x \in A \textrm{ and } x \notin B \}\)
- Symmetric difference: \(A \textrm{ XOR } B := (A \setminus B) \cup (B \setminus A)\)
The following figure illustrates the output of boolean operations on a sphere and a cube:
Boolean Interface¶
PyMesh provides support for all four operations through third party boolean
engines. For example, computing the union of mesh_A and mesh_B can be
achieved with the following snippet:
>>> mesh_A = pymesh.load_mesh("A.obj")
>>> mesh_B = pymesh.load_mesh("B.obj")
>>> output_mesh = pymesh.boolean(mesh_A, mesh_B,
... operation="union",
... engine="igl")
The interface is very minimal and self-explanatory.
The available operations are "union", "intersection", "difference"
and "symmetric_difference". PyMesh supports the following boolean engines:
"igl": Boolean module from libigl, which is also the default engine for 3D inputs."cgal": Naf polyhedron implementation from CGAL."cork": Cork boolean library."carve": Carve boolean library."corefinement": The unpublished boolean engine also from CGAL."clipper": 2D boolean engine for polygons, the default engine for 2D inputs.
The following attributes are defined in the output_mesh:
source: A per-face scalar attribute indicating which input mesh an output face belongs to.source_face: A per-face scalar attribute representing the combined input face index of an output face, where combined input faces are simply the concatenation of faces frommesh_Aandmesh_B.
A Simple Example¶
As a simple example, we are going to operate on the following objects:
- Mesh A (pymesh.ply) contains the extruded text
PyMesh. - Mesh B (plate.ply) contains an extruded wavy plate.
To compute their intersection:
>>> A = pymesh.load_mesh("pymesh.ply")
>>> B = pymesh.load_mesh("plate.ply")
>>> intersection = pymesh.boolean(A, B, "intersection")
>>> # Checking the source attribute
>>> intersection.attribute_names
('source', 'source_face')
>>> intersection.get_attribute("source")
array([ 1., 1., 0., ..., 1., 1., 1.])
The operation is illustrated in the following figure:
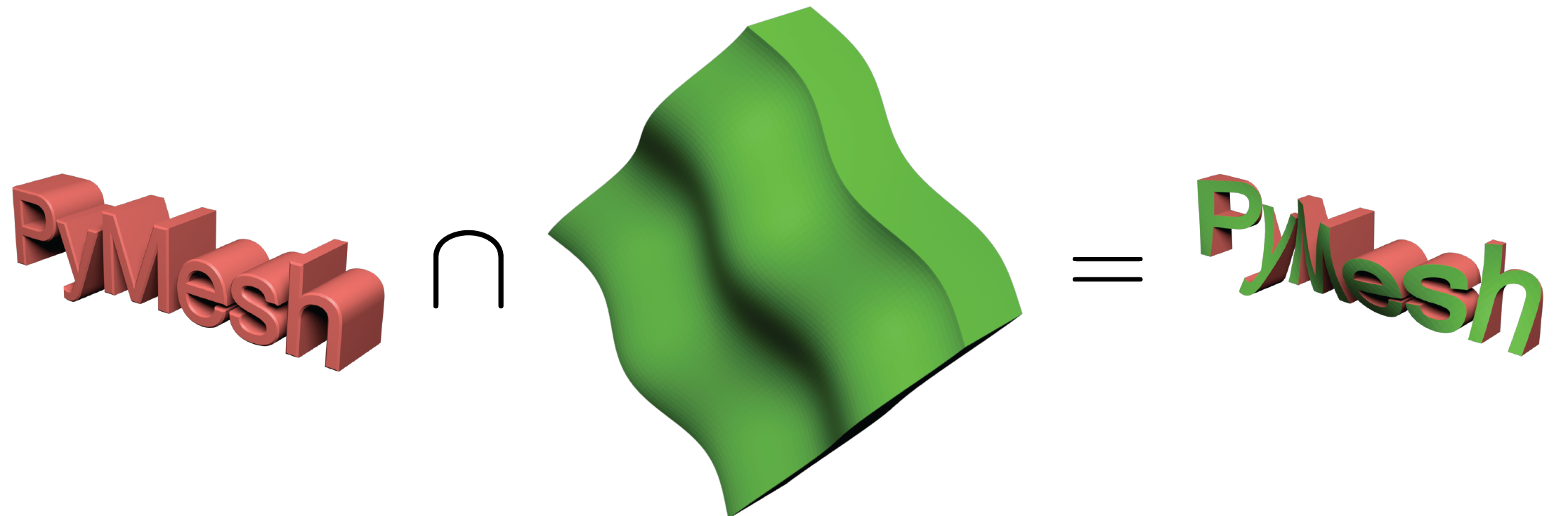
The attribute source tracks the source of each output face. 0 means the
output face comes from the first operand, i.e. pymesh.ply, and 1 means it is from
the second operand, i.e. plate.ply.
The source attribute is useful for assigning the corresponding colors
in the output mesh.
CSG Tree¶
While binary boolean operations are useful, it is often necessary to perform a number of operations in order to create more complex results. A Constructive Solid Geometry tree, aka.CSG tree, is designed for this purpose.
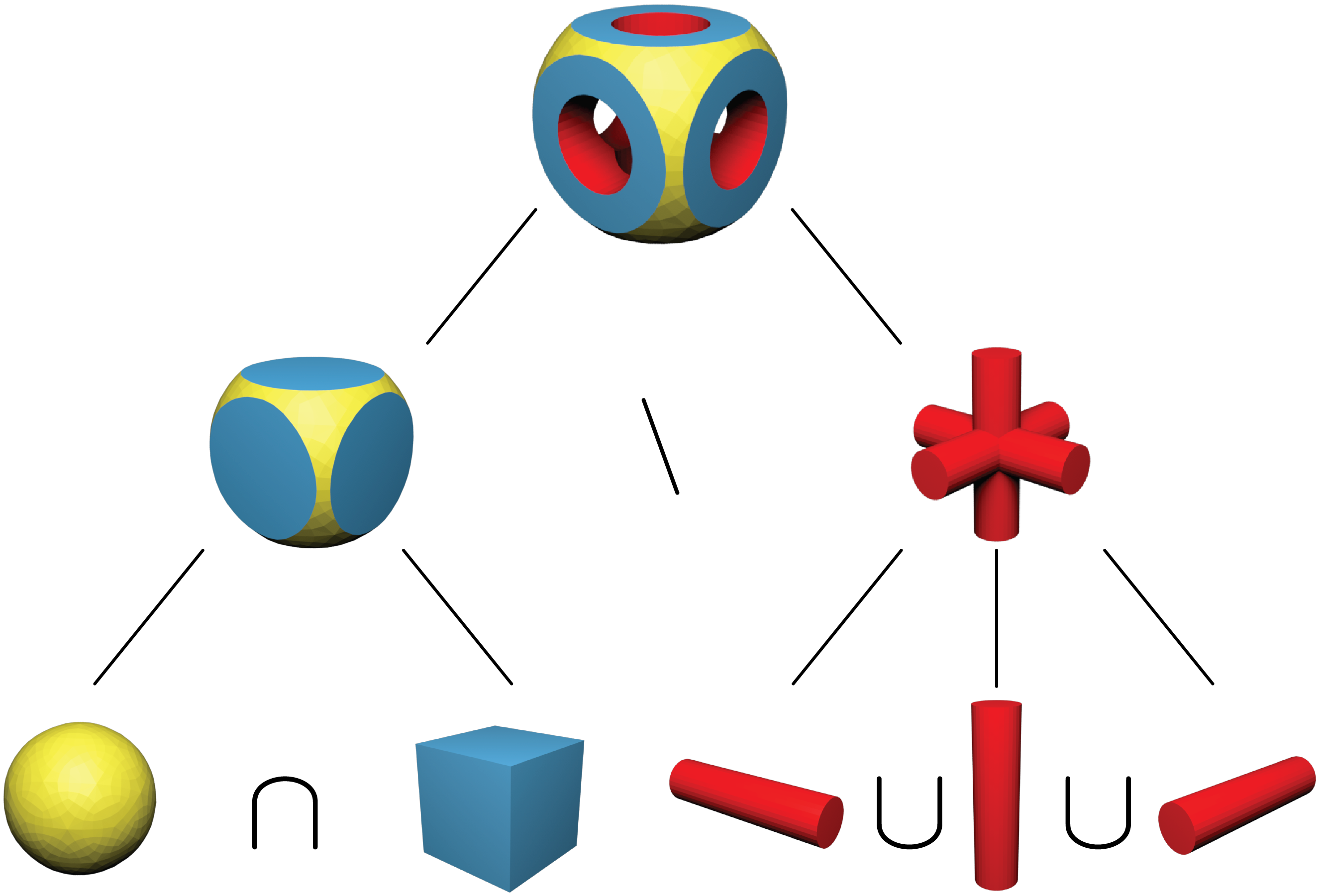
As illustrated in the figure above, CSG tree provides a structured way of
building complex shapes from simple ones. Each node in the tree represents a 3D
shape. Leaf nodes represent user input shapes.
A non-leaf node consists of a boolean operation and a number of child nodes.
The shape it represents can be obtained by performing the specified boolean
operation on shapes from its children. In particular, union and
intersection node can have any number of children (i.e. N-ary union and N-ary
intersection), but difference and symmetric_difference nodes must have
exactly two children.
PyMesh represents CSG tree using pymesh.CSGTree class.
Given the input meshes,
one can construct and evaluate a CST tree using the following code:
>>> ball = pymesh.load_mesh("ball.stl")
>>> box = pymesh.load_mesh("box.stl")
>>> x = pymesh.load_mesh("x.stl")
>>> y = pymesh.load_mesh("y.stl")
>>> z = pymesh.load_mesh("z.stl")
>>> csg = pymesh.CSGTree({
"difference": [
{ "intersection": [{"mesh": box}, {"mesh": ball}] },
{ "union": [{"mesh": x}, {"mesh": y}, {"mesh": z}]}
]
})
>>> output = csg.mesh
Notice that the constructor of CSGTree takes a python dictionary as argument.
The entire tree structure is captured in the dictionary. The context free
grammar for this dictionary is:
Node -> {Operation : Children}
Node -> {"mesh": Mesh}
Node -> CSGTree
Children -> [Node, Node, ...]
Operation -> "union" | "intersection" | "difference" | "symmetric_difference"
where Mesh is a pymesh.Mesh object and CSGTree is a
pymesh.CSGTree object. One can construct the entire tree all together
as shown above or build up the tree incrementally:
>>> left_tree = pymesh.CSGTree({
"intersection": [{"mesh": box}, {"mesh": ball}]
})
>>> right_tree = pymesh.CSGTree({
"union": [{"mesh": x}, {"mesh": y}, {"mesh": z}]
})
>>> csg = pymesh.CSGTree({
"difference": [left_tree, right_tree]
})
>>> left_mesh = left_tree.mesh
>>> right_mesh = right_tree.mesh
>>> output = csg.mesh
